Привет, Хабр! Будущих студентов курса "Алгоритмы и структуры данных" приглашаем на открытый вебинар по теме "Заповедники двоичных деревьев поиска."
А сейчас делимся с вами традиционным переводом полезного материала.
Наихудшая временная сложность таких операций, как поиск, удаление и вставка, для двоичного дерева поиска (Binary Search Tree) составляет O(n). Наихудший случай случай возникает, когда дерево несбалансировано. Мы можем улучшить наихудший результат временной сложности до O(log n) с помощью красно-черных и АВЛ-деревьев.
Можем ли мы добиться на практике лучшего результата, чем тот, что нам дают красно-черные или АВЛ-деревья?
Подобно красно-черным и АВЛ-деревьям, Splay-дерево (или косое дерево) также является самобалансирующимся бинарным деревом поиска. Основная идея splay-дерева состоит в том, чтобы помещать элемент, к которому недавно осуществлялся доступ, в корень дерева, что делает этот элемент, доступным за время порядка O(1) при повторном доступе. Вся суть заключается в том, чтобы использовать концепцию локальности ссылок (в среднестатистическом приложении 80% обращений приходятся на 20% элементов). Представьте себе ситуацию, когда у нас есть миллионы или даже миллиарды ключей, и лишь к некоторым из них обращаются регулярно, что весьма вероятно для многих типичных приложениях.
Все операции со splay-деревом выполняются в среднем за время порядка O(log n), где n - количество элементов в дереве. Любая отдельная операция в худшем случае может занять время порядка Тэта(n).
Операция поиска
Операция поиска в splay-дереве представляет собой стандартный алгоритм поиска в бинарном дереве, после которого дерево выворачивается (искомый узел перемещается в корень — операция splay). Если поиск завершился успехом, то найденный узел поднимается наверх и становится новым корнем. В противном случае корнем становится последний узел, к которому был осуществлен доступ до достижения NULL.
В результате осуществления доступа к узлу возможны следующие случаи:
1. Узел является корневым. Мы просто возвращаем корень, больше ничего не делаем, так как узел, к которому осуществляется доступ, уже является корневым.
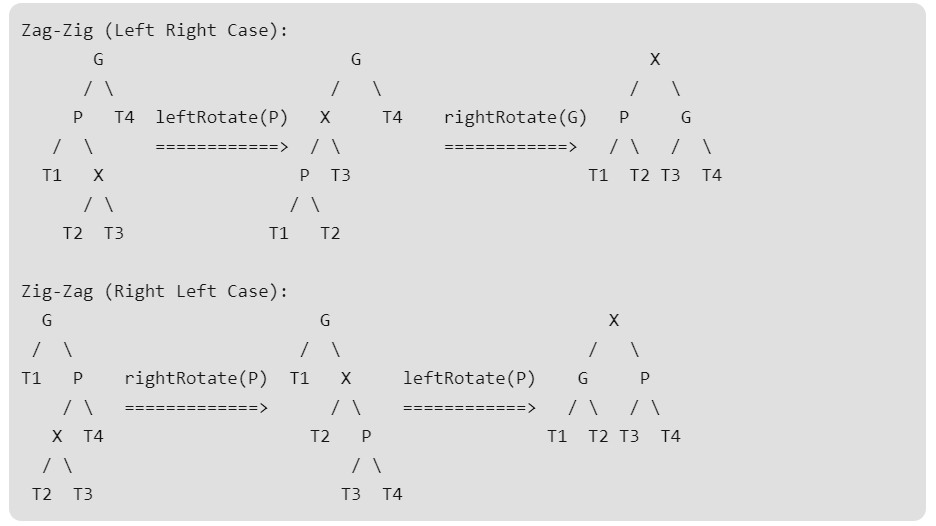
2. Zig: узел является дочерним по отношению к корню (у узла нет прародителя). Узел является либо левым потомком корня (мы делаем правый разворот), либо правым потомком своего родителя (мы делаем левый разворот).
T1, T2 и T3 — поддеревья дерева с корнем y (слева) или x (справа)

3. У узла есть и родитель, и прародитель. Возможны следующие варианты:
а) Zig-Zig и Zag-Zag. Узел является левым потомком родительского элемента, и родитель также является левым потомком прародителя (два разворота вправо) ИЛИ узел является правым потомком своего родительского элемента, и родитель также является правым потомком своего прародитель (два разворота влево).

б) Zig-Zag и Zag-Zig. Узел является левым потомком по отношению к родительскому элементу, а родитель является правым потомком прародителя (разворот влево с последующим разворотом вправо) ИЛИ узел является правым потомком своего родительского элемента, а родитель является левым потомком прародителя (разворот вправо с последующим разворотом влево).
Пример:
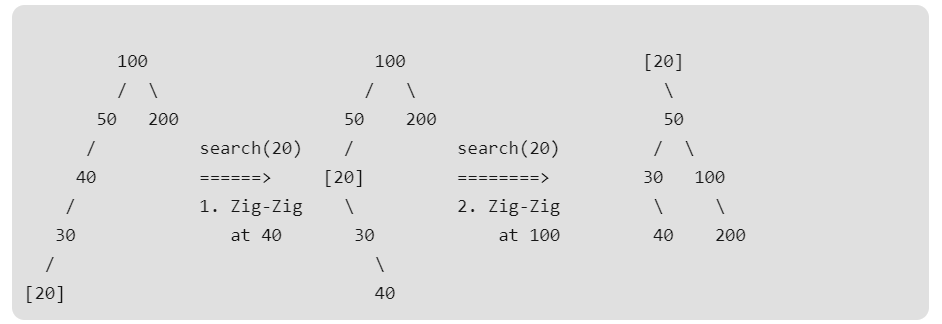
Важно отметить, что операция поиска или разворота (splay) не только переносит найденный ключ в корень, но также уравновешивает дерево. Например в случае выше, высота дерева уменьшается на 1.
Реализации:
C++
#include <bits/stdc++.h>
using namespace std;
// An AVL tree node
class node
{
public:
int key;
node *left, *right;
};
/* Helper function that allocates
a new node with the given key and
NULL left and right pointers. */
node* newNode(int key)
{
node* Node = new node();
Node->key = key;
Node->left = Node->right = NULL;
return (Node);
}
// A utility function to right
// rotate subtree rooted with y
// See the diagram given above.
node *rightRotate(node *x)
{
node *y = x->left;
x->left = y->right;
y->right = x;
return y;
}
// A utility function to left
// rotate subtree rooted with x
// See the diagram given above.
node *leftRotate(node *x)
{
node *y = x->right;
x->right = y->left;
y->left = x;
return y;
}
// This function brings the key at
// root if key is present in tree.
// If key is not present, then it
// brings the last accessed item at
// root. This function modifies the
// tree and returns the new root
node *splay(node *root, int key)
{
// Base cases: root is NULL or
// key is present at root
if (root == NULL || root->key == key)
return root;
// Key lies in left subtree
if (root->key > key)
{
// Key is not in tree, we are done
if (root->left == NULL) return root;
// Zig-Zig (Left Left)
if (root->left->key > key)
{
// First recursively bring the
// key as root of left-left
root->left->left = splay(root->left->left, key);
// Do first rotation for root,
// second rotation is done after else
root = rightRotate(root);
}
else if (root->left->key < key) // Zig-Zag (Left Right)
{
// First recursively bring
// the key as root of left-right
root->left->right = splay(root->left->right, key);
// Do first rotation for root->left
if (root->left->right != NULL)
root->left = leftRotate(root->left);
}
// Do second rotation for root
return (root->left == NULL)? root: rightRotate(root);
}
else // Key lies in right subtree
{
// Key is not in tree, we are done
if (root->right == NULL) return root;
// Zag-Zig (Right Left)
if (root->right->key > key)
{
// Bring the key as root of right-left
root->right->left = splay(root->right->left, key);
// Do first rotation for root->right
if (root->right->left != NULL)
root->right = rightRotate(root->right);
}
else if (root->right->key < key)// Zag-Zag (Right Right)
{
// Bring the key as root of
// right-right and do first rotation
root->right->right = splay(root->right->right, key);
root = leftRotate(root);
}
// Do second rotation for root
return (root->right == NULL)? root: leftRotate(root);
}
}
// The search function for Splay tree.
// Note that this function returns the
// new root of Splay Tree. If key is
// present in tree then, it is moved to root.
node *search(node *root, int key)
{
return splay(root, key);
}
// A utility function to print
// preorder traversal of the tree.
// The function also prints height of every node
void preOrder(node *root)
{
if (root != NULL)
{
cout<<root->key<<" ";
preOrder(root->left);
preOrder(root->right);
}
}
/* Driver code*/
int main()
{
node *root = newNode(100);
root->left = newNode(50);
root->right = newNode(200);
root->left->left = newNode(40);
root->left->left->left = newNode(30);
root->left->left->left->left = newNode(20);
root = search(root, 20);
cout << "Preorder traversal of the modified Splay tree is \n";
preOrder(root);
return 0;
}
// This code is contributed by rathbhupendra C
// The code is adopted from http://goo.gl/SDH9hH
#include<stdio.h>
#include<stdlib.h>
// An AVL tree node
struct node
{
int key;
struct node *left, *right;
};
/* Helper function that allocates a new node with the given key and
NULL left and right pointers. */
struct node* newNode(int key)
{
struct node* node = (struct node*)malloc(sizeof(struct node));
node->key = key;
node->left = node->right = NULL;
return (node);
}
// A utility function to right rotate subtree rooted with y
// See the diagram given above.
struct node *rightRotate(struct node *x)
{
struct node *y = x->left;
x->left = y->right;
y->right = x;
return y;
}
// A utility function to left rotate subtree rooted with x
// See the diagram given above.
struct node *leftRotate(struct node *x)
{
struct node *y = x->right;
x->right = y->left;
y->left = x;
return y;
}
// This function brings the key at root if key is present in tree.
// If key is not present, then it brings the last accessed item at
// root. This function modifies the tree and returns the new root
struct node *splay(struct node *root, int key)
{
// Base cases: root is NULL or key is present at root
if (root == NULL || root->key == key)
return root;
// Key lies in left subtree
if (root->key > key)
{
// Key is not in tree, we are done
if (root->left == NULL) return root;
// Zig-Zig (Left Left)
if (root->left->key > key)
{
// First recursively bring the key as root of left-left
root->left->left = splay(root->left->left, key);
// Do first rotation for root, second rotation is done after else
root = rightRotate(root);
}
else if (root->left->key < key) // Zig-Zag (Left Right)
{
// First recursively bring the key as root of left-right
root->left->right = splay(root->left->right, key);
// Do first rotation for root->left
if (root->left->right != NULL)
root->left = leftRotate(root->left);
}
// Do second rotation for root
return (root->left == NULL)? root: rightRotate(root);
}
else // Key lies in right subtree
{
// Key is not in tree, we are done
if (root->right == NULL) return root;
// Zag-Zig (Right Left)
if (root->right->key > key)
{
// Bring the key as root of right-left
root->right->left = splay(root->right->left, key);
// Do first rotation for root->right
if (root->right->left != NULL)
root->right = rightRotate(root->right);
}
else if (root->right->key < key)// Zag-Zag (Right Right)
{
// Bring the key as root of right-right and do first rotation
root->right->right = splay(root->right->right, key);
root = leftRotate(root);
}
// Do second rotation for root
return (root->right == NULL)? root: leftRotate(root);
}
}
// The search function for Splay tree. Note that this function
// returns the new root of Splay Tree. If key is present in tree
// then, it is moved to root.
struct node *search(struct node *root, int key)
{
return splay(root, key);
}
// A utility function to print preorder traversal of the tree.
// The function also prints height of every node
void preOrder(struct node *root)
{
if (root != NULL)
{
printf("%d ", root->key);
preOrder(root->left);
preOrder(root->right);
}
}
/* Driver program to test above function*/
int main()
{
struct node *root = newNode(100);
root->left = newNode(50);
root->right = newNode(200);
root->left->left = newNode(40);
root->left->left->left = newNode(30);
root->left->left->left->left = newNode(20);
root = search(root, 20);
printf("Preorder traversal of the modified Splay tree is \n");
preOrder(root);
return 0;
}
Java
// Java implementation for above approach
class GFG
{
// An AVL tree node
static class node
{
int key;
node left, right;
};
/* Helper function that allocates
a new node with the given key and
null left and right pointers. */
static node newNode(int key)
{
node Node = new node();
Node.key = key;
Node.left = Node.right = null;
return (Node);
}
// A utility function to right
// rotate subtree rooted with y
// See the diagram given above.
static node rightRotate(node x)
{
node y = x.left;
x.left = y.right;
y.right = x;
return y;
}
// A utility function to left
// rotate subtree rooted with x
// See the diagram given above.
static node leftRotate(node x)
{
node y = x.right;
x.right = y.left;
y.left = x;
return y;
}
// This function brings the key at
// root if key is present in tree.
// If key is not present, then it
// brings the last accessed item at
// root. This function modifies the
// tree and returns the new root
static node splay(node root, int key)
{
// Base cases: root is null or
// key is present at root
if (root == null || root.key == key)
return root;
// Key lies in left subtree
if (root.key > key)
{
// Key is not in tree, we are done
if (root.left == null) return root;
// Zig-Zig (Left Left)
if (root.left.key > key)
{
// First recursively bring the
// key as root of left-left
root.left.left = splay(root.left.left, key);
// Do first rotation for root,
// second rotation is done after else
root = rightRotate(root);
}
else if (root.left.key < key) // Zig-Zag (Left Right)
{
// First recursively bring
// the key as root of left-right
root.left.right = splay(root.left.right, key);
// Do first rotation for root.left
if (root.left.right != null)
root.left = leftRotate(root.left);
}
// Do second rotation for root
return (root.left == null) ?
root : rightRotate(root);
}
else // Key lies in right subtree
{
// Key is not in tree, we are done
if (root.right == null) return root;
// Zag-Zig (Right Left)
if (root.right.key > key)
{
// Bring the key as root of right-left
root.right.left = splay(root.right.left, key);
// Do first rotation for root.right
if (root.right.left != null)
root.right = rightRotate(root.right);
}
else if (root.right.key < key)// Zag-Zag (Right Right)
{
// Bring the key as root of
// right-right and do first rotation
root.right.right = splay(root.right.right, key);
root = leftRotate(root);
}
// Do second rotation for root
return (root.right == null) ?
root : leftRotate(root);
}
}
// The search function for Splay tree.
// Note that this function returns the
// new root of Splay Tree. If key is
// present in tree then, it is moved to root.
static node search(node root, int key)
{
return splay(root, key);
}
// A utility function to print
// preorder traversal of the tree.
// The function also prints height of every node
static void preOrder(node root)
{
if (root != null)
{
System.out.print(root.key + " ");
preOrder(root.left);
preOrder(root.right);
}
}
// Driver code
public static void main(String[] args)
{
node root = newNode(100);
root.left = newNode(50);
root.right = newNode(200);
root.left.left = newNode(40);
root.left.left.left = newNode(30);
root.left.left.left.left = newNode(20);
root = search(root, 20);
System.out.print("Preorder traversal of the" +
" modified Splay tree is \n");
preOrder(root);
}
}
// This code is contributed by 29AjayKumar
C#
// C# implementation for above approach
using System;
class GFG
{
// An AVL tree node
public class node
{
public int key;
public node left, right;
};
/* Helper function that allocates
a new node with the given key and
null left and right pointers. */
static node newNode(int key)
{
node Node = new node();
Node.key = key;
Node.left = Node.right = null;
return (Node);
}
// A utility function to right
// rotate subtree rooted with y
// See the diagram given above.
static node rightRotate(node x)
{
node y = x.left;
x.left = y.right;
y.right = x;
return y;
}
// A utility function to left
// rotate subtree rooted with x
// See the diagram given above.
static node leftRotate(node x)
{
node y = x.right;
x.right = y.left;
y.left = x;
return y;
}
// This function brings the key at
// root if key is present in tree.
// If key is not present, then it
// brings the last accessed item at
// root. This function modifies the
// tree and returns the new root
static node splay(node root, int key)
{
// Base cases: root is null or
// key is present at root
if (root == null || root.key == key)
return root;
// Key lies in left subtree
if (root.key > key)
{
// Key is not in tree, we are done
if (root.left == null) return root;
// Zig-Zig (Left Left)
if (root.left.key > key)
{
// First recursively bring the
// key as root of left-left
root.left.left = splay(root.left.left, key);
// Do first rotation for root,
// second rotation is done after else
root = rightRotate(root);
}
else if (root.left.key < key) // Zig-Zag (Left Right)
{
// First recursively bring
// the key as root of left-right
root.left.right = splay(root.left.right, key);
// Do first rotation for root.left
if (root.left.right != null)
root.left = leftRotate(root.left);
}
// Do second rotation for root
return (root.left == null) ?
root : rightRotate(root);
}
else // Key lies in right subtree
{
// Key is not in tree, we are done
if (root.right == null) return root;
// Zag-Zig (Right Left)
if (root.right.key > key)
{
// Bring the key as root of right-left
root.right.left = splay(root.right.left, key);
// Do first rotation for root.right
if (root.right.left != null)
root.right = rightRotate(root.right);
}
else if (root.right.key < key)// Zag-Zag (Right Right)
{
// Bring the key as root of
// right-right and do first rotation
root.right.right = splay(root.right.right, key);
root = leftRotate(root);
}
// Do second rotation for root
return (root.right == null) ?
root : leftRotate(root);
}
}
// The search function for Splay tree.
// Note that this function returns the
// new root of Splay Tree. If key is
// present in tree then, it is moved to root.
static node search(node root, int key)
{
return splay(root, key);
}
// A utility function to print
// preorder traversal of the tree.
// The function also prints height of every node
static void preOrder(node root)
{
if (root != null)
{
Console.Write(root.key + " ");
preOrder(root.left);
preOrder(root.right);
}
}
// Driver code
public static void Main(String[] args)
{
node root = newNode(100);
root.left = newNode(50);
root.right = newNode(200);
root.left.left = newNode(40);
root.left.left.left = newNode(30);
root.left.left.left.left = newNode(20);
root = search(root, 20);
Console.Write("Preorder traversal of the" +
" modified Splay tree is \n");
preOrder(root);
}
}
// This code is contributed by 29AjayKumar Выходные данные:
Preorder traversal of the modified Splay tree is 20 50 30 40 100 200
Резюме
1) Splay-деревья обладают отличным свойством локальности. Часто используемые элементы легко найти. Редкие элементы не мешаются при поиске.
2) Все операции со splay-деревом в среднем занимают время порядка O(log n). Можно строго доказать, что Splay-деревья работают в среднем за время порядка O(log n) на операцию при любой последовательности операций (при условии, что мы начинаем с пустого дерева)
3) Splay-деревья проще по сравнению с красно-черными и АВЛ-деревьями, так как узлы splay-дерева не требуют дополнительных полей.
4) В отличие от АВЛ-дерева, splay-дерево может изменяться даже при выполнении операций чтения, таких как поиск.
Применение Splay-деревьев
Splay-деревья стали наиболее широко используемой базовой структурой данных, изобретенной за последние 30 лет, потому что они являются самым быстрым типом сбалансированного дерева поиска для огромного множества приложений.
Splay-деревья используются в Windows NT (в виртуальной памяти, сети и коде файловой системы), компиляторе gcc и библиотеке GNU C++, редакторе строк sed, сетевых маршрутизаторах Fore Systems, наиболее популярной реализации Unix malloc, загружаемых модулях ядра Linux и во многих других программах (Источник: http://www.cs.berkeley.edu/~jrs/61b/lec/36)
Смотрите также Splay Tree | Set 2 (Insert).
Ссылки:
http://www.cs.berkeley.edu/~jrs/61b/lec/36
http://www.cs.cornell.edu/courses/cs3110/2009fa/recitations/rec-splay.html
http://courses.cs.washington.edu/courses/cse326/01au/lectures/SplayTrees.ppt
Узнать подробнее о курсе "Алгоритмы и структуры данных".
Записаться на открытый вебинар по теме "Заповедники двоичных деревьев поиска."
Реклама которая может быть полезна
Прямо сейчас в OTUS действуют максимальные новогодние скидки на все курсы. Ознакомиться с полным списком курсов вы можете по ссылке ниже. Также у всех желающих есть уникальная возможность отправить адресату подарочный сертификат на обучение в OTUS.
Кстати, о "красивой упаковке" онлайн-сертификатов мы рассказываем в этой статье.



kovserg
1. Не понятно как достигается сбалансированность например если мы хоти взять минимальный и максимальный элемент в дереве или постоянно ищем около этих узлов.
2. Что мешает использовать например 2 сбалансированных дерева для горячих 20% и остальных холодных 80%. И просто регулировать долю и управлять переносом из одного дерева в другое плюс деревья гарантировано сбалансированы.
3. А где собственно тесты производительности?